Construct Congruent Angles

Today we’ll be looking at another basic construction: congruent angles.  You can use this construction to copy any angle without using a protractor to measure.

1. Begin with the angle you would like to copy.
2. Draw any ray.  This will act as the baseline for your new angle.  The vertex (V) of your original angle will correspond with the endpoint (V1) of your new ray.
3. Set your compass point on V and draw an arc with the radius set to any length.
4. Extend that arc so that it crosses both rays of angle V.
5. Keeping the same compass opening, draw a matching arc with V1 as its center.

 

Notice how in our original angle, we now have three points (V, A, and B) — the vertex and the two points where our arc crosses the rays of the angle.  These three points (like any three points on a plane) form a triangle. This construction makes use of the geometric theorem that states that if two triangles have all three sets of sides congruent, their three angles are also congruent (frequently called the Side-Side-Side Theorem).

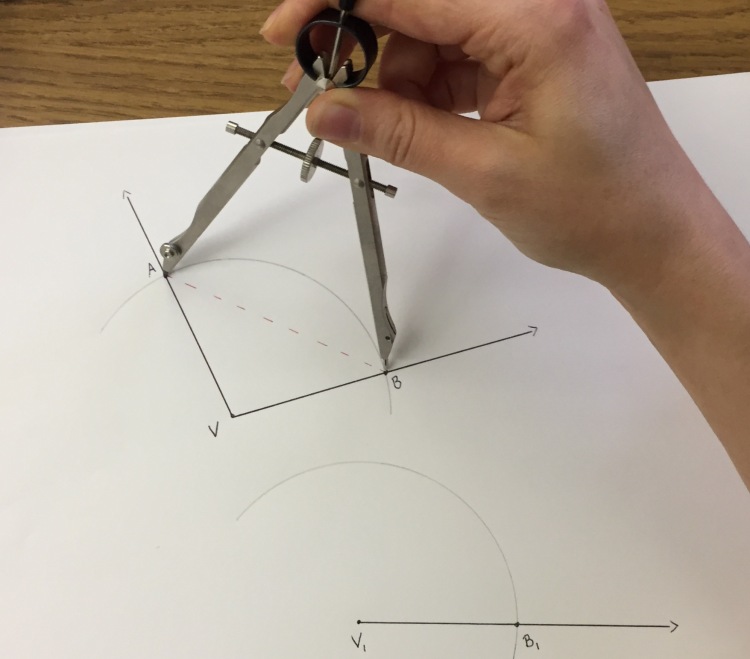
6. Set your compass so that one point is on B and the other on A.

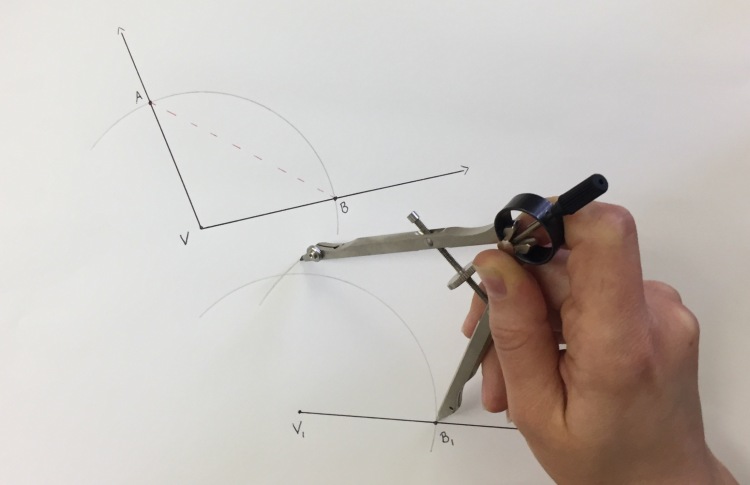7. Keeping the same opening, transfer your compass to Point B1 and draw an arc.

 

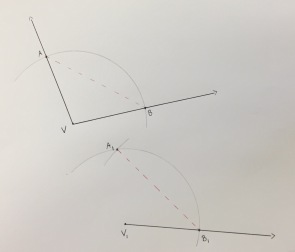
8. The point where these two arcs cross corresponds with Point A, so we’ll call it A1.

 

 

 

 

 

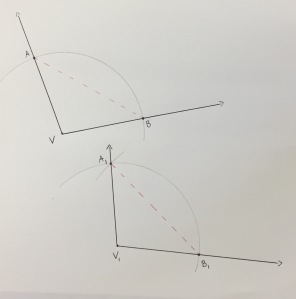
9. Draw the ray from the vertex through A1 to complete your angle.

Notice that, because they are radii of the same size circle, VA, VB, V1A1, and V1B1 are all congruent. For the same reason, AB and A1B1 are congruent. Thus we have two triangles with all three corresponding sides congruent and, according to the Side-Side-Side Theorem, angles V and V1 must also be congruent!